Which Of The Following Has A Radius (Linear Size) Closest To That Of A Neutron Star?
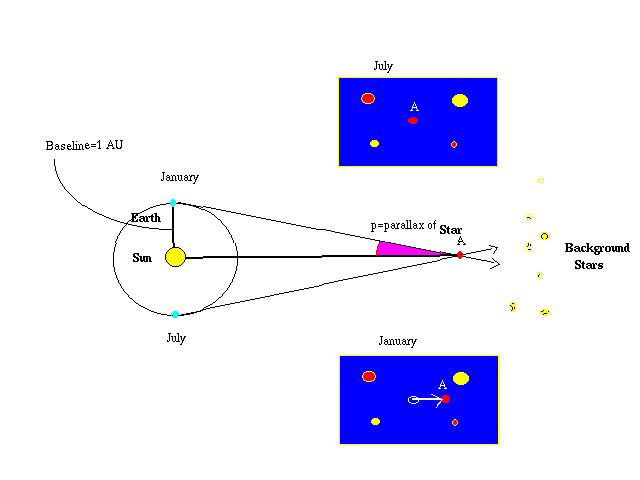
Schematic for computing the parallax of a star.
Why is information technology so hard to figure out the sizes and distances of celestial objects? Part of the reason is psychological. Humans have tended to regard themselves as the pinnacle of creation and the centre of the universe. So there is resistance to the notion that the heaven may comprise objects much larger than the Earth. Throughout history, we have slowly but steadily realized that the Globe is only a tiny role of a vast universe. The other reason is practical. As the Greeks knew, it is well-nigh impossible to measure out the linear size of a distant unfamiliar object directly past eye. We tin can really merely specify its angular size.
Linear size and linear altitude are hard to judge. People sometimes study an unfamiliar object in the heaven and say something like "It looked every bit big as a dinner plate," but this argument conveys almost no useful data. When a bright shooting star or fireball is seen in the heaven, astronomers often receive reports that "Information technology looked close; it landed just over the colina." These reports are nearly always incorrect. Fireballs are typically in the upper atmosphere, 60 to 100 miles from the observers. To utilise angular measurement and say the fireball looked ½° across, "the aforementioned size as the Moon" would be right; but to unconsciously catechumen to linear measurement and say "It was as big equally an aeroplane" is probable to be incorrect if the object itself is unfamiliar.
The ancient Greeks invented trigonometry, which allows quantitative relationships between angles, linear sizes, and linear distances. Through simple mathematical relations, involving basic trigonometry, we can calculate distances of remote objects whose sizes are known (or sizes, if the distances are known).
Angles and linear measures tin exist combined in an extremely useful and unproblematic equation called the small-scale-angle equation. This equation involves the athwart size of an object, its linear size, and its altitude. If whatsoever two of these quantities are known, the third tin can be calculated. Let us refer to the athwart size with the symbol a, expressed in seconds of arc. Allow us refer to the diameter of the object as d and its distance as D. Then the small-angle equation is
a / 206,265 = d / D
A chart for the conversion between degrees and radians, forth with the signs of the major trigonometric functions in each quadrant.
The number 206,265 is called a abiding of proportionality; information technology stays the aforementioned in all applications of the equation. The number 206,265 is really the number of seconds of arc in an angle of 57.3°, which is a special bending called a radian. A radian is defined as an angle subtending i radius of a circle, laid along the circumference of the circle. Since the circumference of a circumvolve is 2πr, a radian is 360° / ii π =57.3° or almost a sixth of a total circumvolve. It is an of import angle with many applications in geometry.
Hither is an example of the use of the pocket-sized bending equation. Suppose a friend who is 2 meters alpine is standing beyond a field from you lot, where he or she subtends an angle of ½°, or 1800". How far away are they? Nosotros want to solve the equation for D. Rearranging the equation, nosotros have
D = 206,265 d / a
Using metric units, we would have
D = (2.1 x 105 ten 2) / i.viii 10 10iii = 2.3 x 102 meters = 230 meters
If your friend is 2 meters tall and subtends an bending of ½° (or 1800 arc seconds), their distance D is 230 meters. In other words, your friend is about one-third of a mile away. Notice nosotros are rounding off all our estimates to 2 significant figures because the bending measurement is not likely to be very accurate.
Equally the Greeks realized, the small-scale-angle equation can exist used to investigate astronomical distances. They could not mensurate the Moon'southward diameter accurately, but they knew its angular size a, which is also roughly ½°, or 1800". If we use the modern noesis that the Moon is nigh 3,500 kilometers in diameter, we can approximate its distance just equally we did for the friend's distance above. In metric units, d would be 3.5 × x6 meters. The equation would read
D = (2.one × 105 × three.5 × 10vi) / (ane.8 × 103) ? 4 10 108 meters ? 4 x 105 kilometers
This is about 400,000 kilometers. Notice one time again the symbol "?" meaning "approximately equal to." It is useful whenever judge values such as one° are involved. In other words, the measurement of athwart size is gauge, so the resulting estimate of distance must also be approximate.
Here is another case of the apply of the small bending equation: How large are the smallest craters that we tin see on the Moon with a lawn telescope? To solve this nosotros first with the information that a expert lawn telescope can resolve or make out angular particular as small-scale equally i second of arc, or 1". The moon is 384,000 kilometers abroad. And then nosotros are asking how big is an object that subtends i" at that distance. Rearranging the small angle equation again
d = aD / 206,265
Plugging in the Moon's altitude and the angle of one second of arc
d = (1 x iii.viii 10 105) / (two.1 ten 105) = one.8 kilometers
We can see features as minor equally a mile beyond on the Moon with a telescope. The eye can only make out angular scales of virtually 3 minutes of arc. Then when you lot stare at the Moon, the smallest features yous can see are 3 × 60 × one.8? 320 kilometers or about 200 miles across.
Finally, if the Sun has a diameter of virtually one.4 million kilometers and is 150 meg kilometers abroad (too called one Astronomical Unit or 1 A.U.), what bending does the Lord's day subtend in the sky? We need to solve the small-angle equation for a, so rearranging it ane last time
a = (d / D) 10 206,265
Substituting the values for bore d and distance D, we become
a = (1.4 ten ten6 / 1.five ten 108) x (2.ane x 10five) = 1960"
Since there are 3600" in one degree, this angle is 1960 / 3600 = 0.54°. The Sun and the Moon differ greatly in size and in altitude from the Earth. Information technology is because of the coincidence that they both subtend the same angle of ½° that eclipses are possible.
Which Of The Following Has A Radius (Linear Size) Closest To That Of A Neutron Star?,
Source: https://www.teachastronomy.com/textbook/Early-Astronomy/Angular-Size-and-Linear-Size/
Posted by: shafferwhow1970.blogspot.com


0 Response to "Which Of The Following Has A Radius (Linear Size) Closest To That Of A Neutron Star?"
Post a Comment